 Shoemaker resume ahora todo esto, de manera
compacta y clara:
Shoemaker resume ahora todo esto, de manera
compacta y clara:
 que resume bastante bien su naturaleza y su uso.
Wikipedia ilustra sobre el tema en
https://en.wikipedia.org/wiki/Quaternions_and_spatial_rotation,
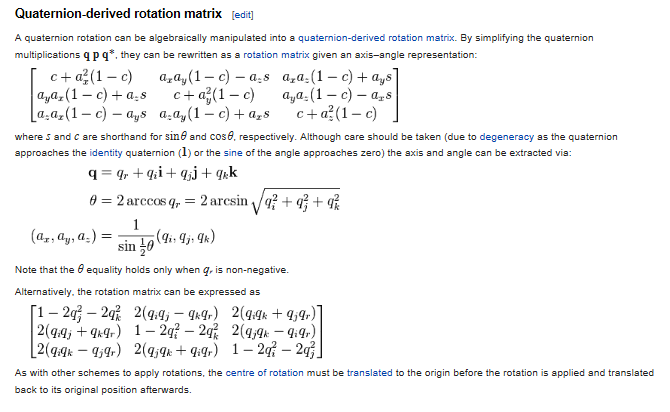
en particular el paso de quaterniones a la
matriz equivalente.
que resume bastante bien su naturaleza y su uso.
Wikipedia ilustra sobre el tema en
https://en.wikipedia.org/wiki/Quaternions_and_spatial_rotation,
en particular el paso de quaterniones a la
matriz equivalente.
 Vea unas reflexiones propias sobre
Matrices en espacio vectorial 3D. Y algunas intuiciones geométricas de esos
entes, en
Movimientos y transformaciones en plano y espacio. Descripción geométrica.
En VisualStudio podemos encontrar la matriz
correspondiente a un quaternion mediante la operación Matrix3D.Rotate(Quaternion),
aplicada a una matriz identidad (Identity). Después podemos rotar puntos y
vectores mediante esa matriz.
Y aqui vemos cómo rotar puntos y/o vectores
mediante quaterniones. Puntos y vectores han de pasarse a quaterniones para
poder multiplicarlos mediante producto matricial
.
Vea unas reflexiones propias sobre
Matrices en espacio vectorial 3D. Y algunas intuiciones geométricas de esos
entes, en
Movimientos y transformaciones en plano y espacio. Descripción geométrica.
En VisualStudio podemos encontrar la matriz
correspondiente a un quaternion mediante la operación Matrix3D.Rotate(Quaternion),
aplicada a una matriz identidad (Identity). Después podemos rotar puntos y
vectores mediante esa matriz.
Y aqui vemos cómo rotar puntos y/o vectores
mediante quaterniones. Puntos y vectores han de pasarse a quaterniones para
poder multiplicarlos mediante producto matricial
.
Rotating PointsProbably the most important result on this page is the formula for representing rotations in 3 dimensions using quaternions (although we will also discuss how to use quaternions for other transforms on this page). This formula for 3D rotations is: Pout = q * Pin * conj(q) where:
q = cos(a/2) + i ( x * sin(a/2)) + j (y * sin(a/2)) + k ( z * sin(a/2)) where:
So why is the rotation represented by this strange formula where the vector to be transformed is 'sandwiched' between two slightly different variants of 'q'? It is quite hard to get an intuitive grasp of this, although its easy to use it if you don't worry too much about how it works. If, like me, you like to know why things work then I have tried to work out an answer on this page.
|
| http://www.euclideanspace.com/maths/algebra/realNormedAlgebra/quaternions/transforms/index.htm |